Solve the system of equations by using the method of cross multiplication x 2y 1 = 0, 2x – 3y – 12 = 0 asked 6 days ago in Linear Equations by Hailley (260k points) linear equations in two variables;Unlock StepbyStep (x^2y^21)^3x^2y^3=0 Extended Keyboard ExamplesQuestion I am solving by using the elimination method 5xy=13 6x5y=8 5x=y 3x4y=18 1/2x3y=11 2xy=5 3x2y=2 x3y=4 3xy=6 x2y=2 4xy=1 x2y=7 2x5y=1 3x4y=5 Answer by MathLover1() (Show Source)
How To Solve Using The Gauss Elimination Method Y Z 2 2x 3z 5 X Y Z 3 Quora
5/x-3/y=1 3/2x 2/3y=5 by elimination method
5/x-3/y=1 3/2x 2/3y=5 by elimination method- By solving this equation by eliminating method y and y would get canceled, leaving 2x 3x = 5 10 5x = 15 x = 3 By putting value of x in equation 1, 2x y = 5 2 × 5 y = 5 y = 10 5 y = 5 Answer link Related questions How do you solve systems of equations by elimination using multiplication?Question 1 Solve the following pair of linear equations by the elimination method and the substitution method x y =5 and 2x –3y = 4 3x 4y = 10 and 2x – 2y = 2 3x – 5y – 4 = 0 and 9x = 2y 7 x/2 2y /3 = 1 and x – y/3 = 3



What Is The Value Of X And Y 2x 3y 5 X 2y 1 Quora
You can put this solution on YOUR website!(a) 2x 3y = 12(i) and x y = 1(ii) (ii)×3 ==> 3x 3y = 3(iii) Now we can eliminate y by adding (i) & (iii) (i) (iii) ==> 5x = 15 so x=3Steps for Solving Linear Equation 5x3y = 2 5 x 3 y = 2 Subtract 3y from both sides Subtract 3 y from both sides 5x=23y 5 x = 2 − 3 y Divide both sides by 5 Divide both sides by 5
Substitution for this system of equations will be complicated because we will have to work with fractions, so let's try elimination method again $$\begin{align} 2x 3y &= 3 \\02cm 5x 2yExample Two Elimination by Subtracting Solve these equations by elimination 5y 7x = 8 3y 7x = 2 Answer Remember that subtracting the second equation means that all signs of the second equation change to their opposite signs 5y 7x = 8 – (3y 7x = 2) 2y = 6Solution for 2x3y=5 equation Simplifying 2x 3y = 5 Solving 2x 3y = 5 Solving for variable 'x' Move all terms containing x to the left, all other terms to the right
Solve using the elimination method Show your work If the system has no solution or an infinite number of solutions, state this 2x 6y = 12 x 3y = 3 3 Solve using the elimination method Show read more Ex 46, 11 Solve system of linear equations, using matrix method 2x y z = 1 x – 2y – z = 3/2 3y – 5z = 9 The system of equation is 2x y z = 1 x – 2y – z = 3/2 3y – 5z = 9 Writing above equation as AX = B 8(2&1&1@1&−2&−1@0&3&−5) 8(𝑥@𝑦@𝑧 Step 1 Label the equations Our equations were 3x y = and 2x 2y = 16 Label the equations A and B (A) 3x y = (B) 2x 2y = 16 Step 2 Isolate one of the variables Next, we need to isolate one of the variables We will isolate variable y in equation A in this solution Equation A is 3x y =



Algebra Ppt Download



Solve The Following Systems Of Equations 1 7x 1 6y 3 1 2x 1 3y 5 Sarthaks Econnect Largest Online Education Community
Solve the following system using the elimination method 6x 2y = 5 12x 4y = Algebra I have two equations that I need to solve using the elimination method the system is x = y = 9 and 2x y = 3 Math Use the elimination method to solve the system of equations 5u2v=15 3uv=7 x 2y 5 3x 2 3y 10 solve by elimination method Mathematics TopperLearningcom dzs9yv22 Starting early can help you score better!2/3x3/5y=17 1/2x1/3y=1 Rewrite each equation by multiplying the LCD of each equation respectively 10x 9y = 255 3x 2y = 6 Use elimination method to solve this system



7 4x 3 Y 2x 3y 1 0 Mathematics Topperlearning Com Iwb1egg
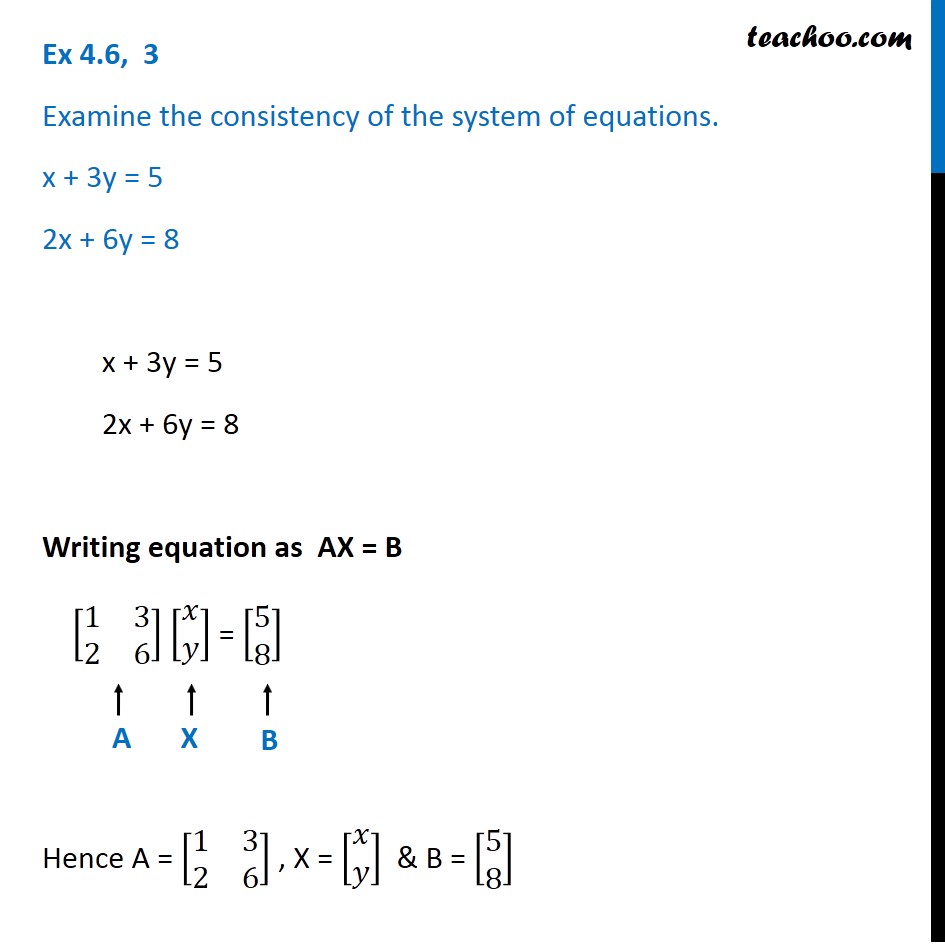



Ex 4 6 3 Examine Consistency X 3y 5 2x 6y 8 Ex 4 6
2(2) 3y = 5 3y = 9 y = 3 or5(2) 2y = 4 10 2y = 4 2y = 6 y = 3 Alternate Procedure eliminate x The coefficients of x are not the same in the two equations but if they were it would possible to add the two equations and the y terms would cancel out2 algebraic methods (elimination and substitution) and graphical method Elimination 2x 3y = 5 So 6x 9y = 15 (equation 1) 3x y = 4 6x 2y = 8 (equation 2) (6x 9y) (6x 2y) = 15 8 7y = 7 y = 1 (equation 3) Substitute y = 1 into equati0 votes 1 answer Solve for x and y 5/x 3/y = 1 , 3/2x 2/3y = 5 asked Jun 22 in Linear Equations by Gavya (251k




3 58 Solve The System By Using The Elimination Chegg Com




Ex 3 4qno1 1 Solve X Y 5 2x 3y 4 By The Elimination Method Youtube
Click here 👆 to get an answer to your question ️ 3/2x 2/3y = 5 , 5/x 3/y = 1 Solve by Elemination methodAnswer only if you knowCan any system be solved using theClick here👆to get an answer to your question ️ Solve by elimination method x y = 5 2x 3y = 4 Join / Login > 10th > Maths > Pair of Linear Equations in Two Variables > Algebraic Methods of Solving a Pair of Linear Equations
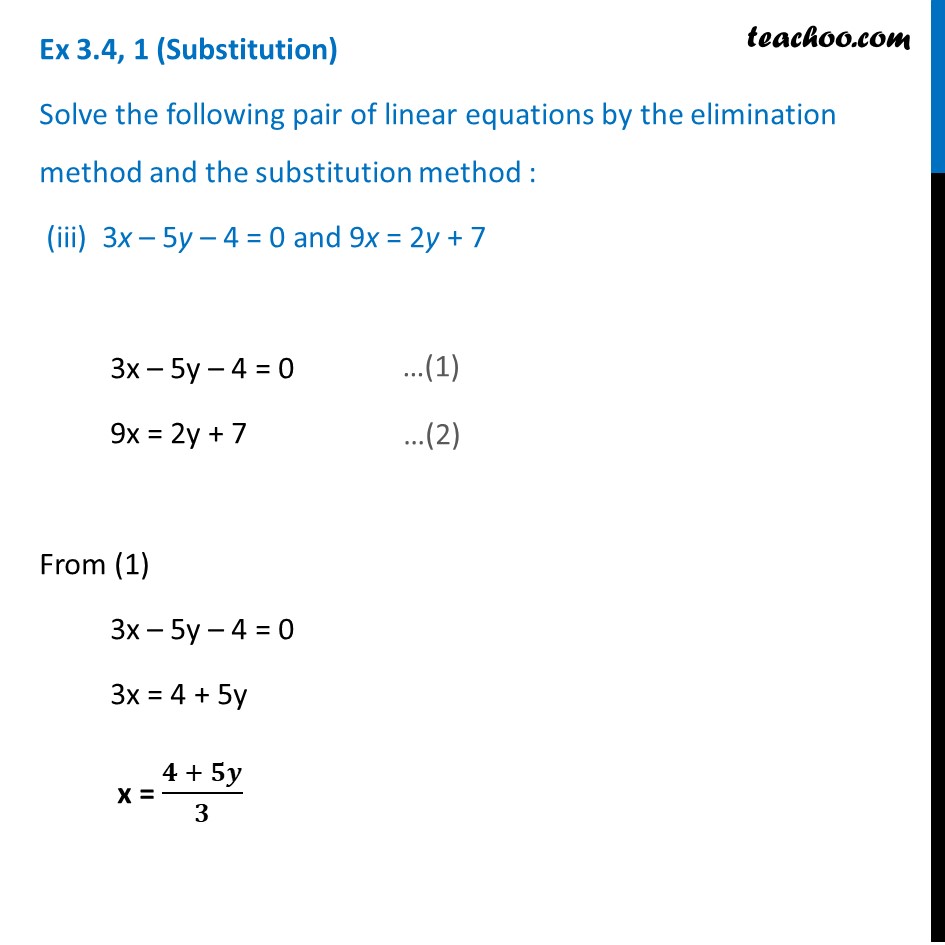



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y




4x 3y 1 And 5x 7y 2 Solve For X Using Elimination Method Brainly In
Free system of equations calculator solve system of equations stepbystep Now multiply equation (1) by 5 and (2) by 7 By adding both the equations Substitute the value of x in equation (1) Therefore, x = 7 and y = 2 If x = 7 and y – 2 satisfy the equation (3) then we can say that the equations hold simultaneously Substitute the value of x and y in equation (3) 43 = 43 which is trueQuestion 1 Solve the following systems of linear equations by Gaussian elimination method 2x − 2y 3z = 2, x 2y − z = 3, 3x − y 2z = 1



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y



Cbse 10 Math Cbse Linear Equations In Two Variables Ncert Solutions
Chapter 4 of the RBSE Class 9 Maths will help the students to solve the given pair of linear equations using different methods After practicing these important questions, students will be able to understand the graphical method of solving, various algebraic methods of solving the given system of equations Integrate 12x 2 (32x) 5 Take (32x) 5 = (3 2x) 2 (3 2x) 3 Principal algebraic expressions and formulas (ab) 2 = a 2 2abb 2 and (ab) 3 =a 3 3a 2 b3ab 2 b 3 = (9 4x 2 12x)(27 8x 3 54x 18x ) FOIL method the product of two binomials is the sum of the products of the First terms, the Outer terms, the Inner terms and the Last terms That would be the easiest solving method to use here Set up the problems like this, add them, and solve for the remaining variable x 3y = 5 x 3y = 12x = 4 x = 2 Now that you know what x is, you can substitute this value for x in one of the equations x 3y = 5 2 3y = 5 3y = 3 y = 1 The solution to this system would be (2, 1)




Ml Aggarwal Solutions For Class 9 Maths Chapter 5 Simultaneous Linear Equations




The Value Of K For Which The System Of Equations 2x 3y 5
Solve by elimination method x y = 8/3 2x/5 y = 7/5 Maths Simultaneous Linear EquationsSolve by Addition/Elimination 5x2y=3 , 2xy=0 5x − 2y = 3 5 x 2 y = 3 , 2x − y = 0 2 x y = 0 Multiply each equation by the value that makes the coefficients of y y opposite 5x−2y = 3 5 x 2 y = 3 (−2)⋅(2x−y) = (−2)(0) ( 2) ⋅ ( 2 x y) = ( 2) ( 0) Simplify Tap for more steps Simplify (Simple and best practice solution for 3(y5)2=5 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand,




Solve The Following System Of Equation 2x 3y 8 0 4x 5y 14 0



What Is The Answer To Find The Equation Of A Straight Line Through The Point Of Intersection Of Lines 2x 3y 5 0 And 3x 4y 7 0 Which Is Parallel To The Line 2x Y 2 0 Quora
Use elimination to solve each system of equations xy=3 2x3y=16 Algebra Solve the system by the elimination method 6x2y=16 x3y=16 Algebra Solve using the elimination methodShow workIf the system has no solution or an infinite number of solutions state this 7x8y=51,7x10y=55 algebra q solve x y 3 and 2x 5y 10 using elimination method Mathematics TopperLearningcom 0yujch00 Solve using the elimination method Show your work If the system has no solution or an infinite number of solutions, state this 2x 6y = 12 x 3y = 3 3 Solve using the elimination method Show read more
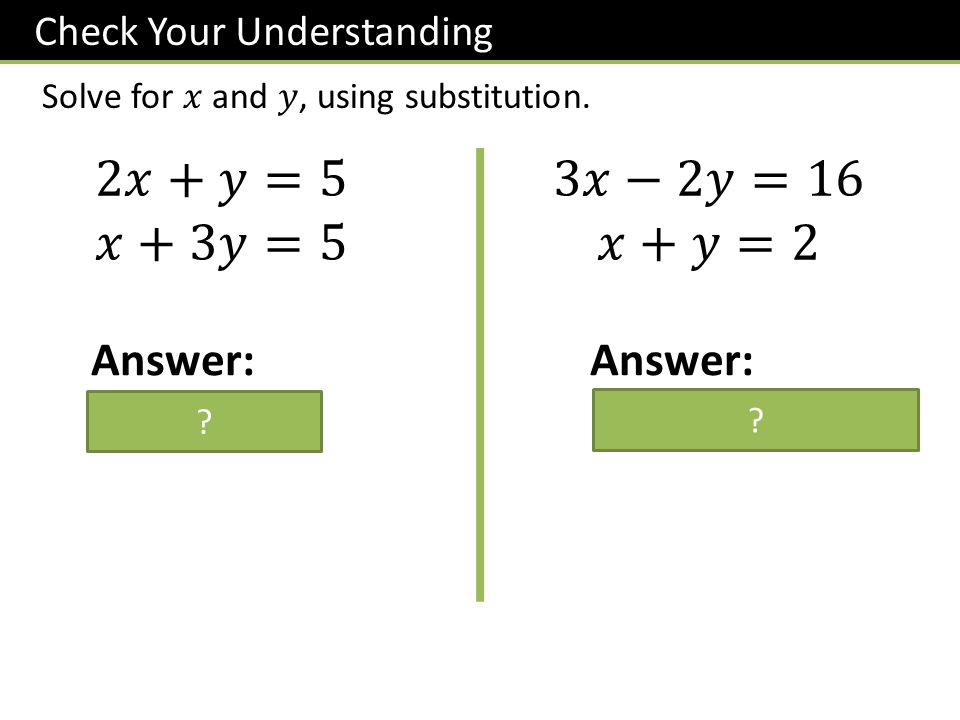



Year 9 Simultaneous Equations Ppt Video Online Download




4x Y 3 8 3 And X 2 3y 4 5 2 Solve By Elimination Method Maths Pair Of Linear Equations In Two Variables Meritnation Com
using Gaussian or GaussJordan Elimination x y z = 5 2x – 3y 6z = 32 4x 5y 10z = 8 asked in ALGEBRA 2 by anonymous gaussjordanmethodSolving system of equation by substitution method, involves solving any one of the given equation for either 'x' or 'y' and plugging that in the other equation and solve that equation for another variableSubstitution method questions 2 Step 1 Solve any one of the equations either xAvail 25% off on study pack




Solve The Following Simultaneous Equation By Elimination Method 3x 4y 10 4x 3y 5
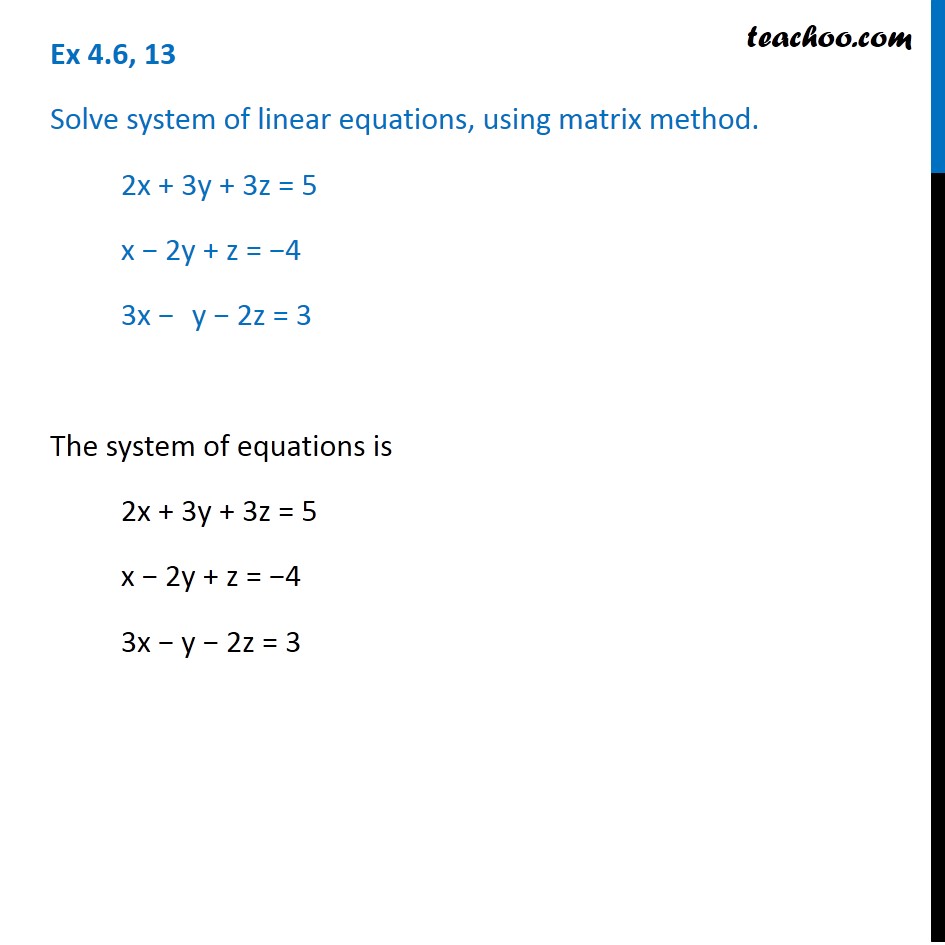



Ex 4 6 13 Solve Linear Equations Using Matrix Method Ex 4 6
The given equations are `(3)/(2x) (2)/(3y) = 5` and `(5)/x (3)/y` = 1 Let `(1)/x = "a" and (1)/y = "b"` Then, we have `(3)/(2)"a" (2)/(3)"b"` = 5Solve using Elimination Method3x 5y 4 = 09x = 2y 7Or click the example
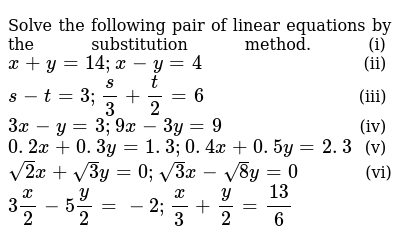



Ncert Solutions For Class 10 Maths Pair Of Linear Equations In Two Variables



What Is The Value Of X And Y 2x 3y 5 X 2y 1 Quora
Solve the following systems of simultaneous linear equations by the elimination method (1 to 9) 1 (i) 3x 4y = 10 2x – 2y = 2 (ii) 2x = 5y 4 3x – 2y 16 = 0 Solution (i) 3x 4y = 10 (1) 2x – 2y = 2 (2) Multiplying equation (1) by 1 and (2) by 2 3x 4y = 10 4x – 4y = 4 By adding both the equations 7x = 14 ByExample (Click to try) xy=5;x2y=7 Try it now Enter your equations separated by a comma in the box, and press Calculate!Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!




7 Solve The Following System Of Linear Equations Chegg Com




5 X 3 Y 1 3 2x 2 3y 5 Find The Value Of X Y By Substitution Method To Be A Brainliest Brainly In
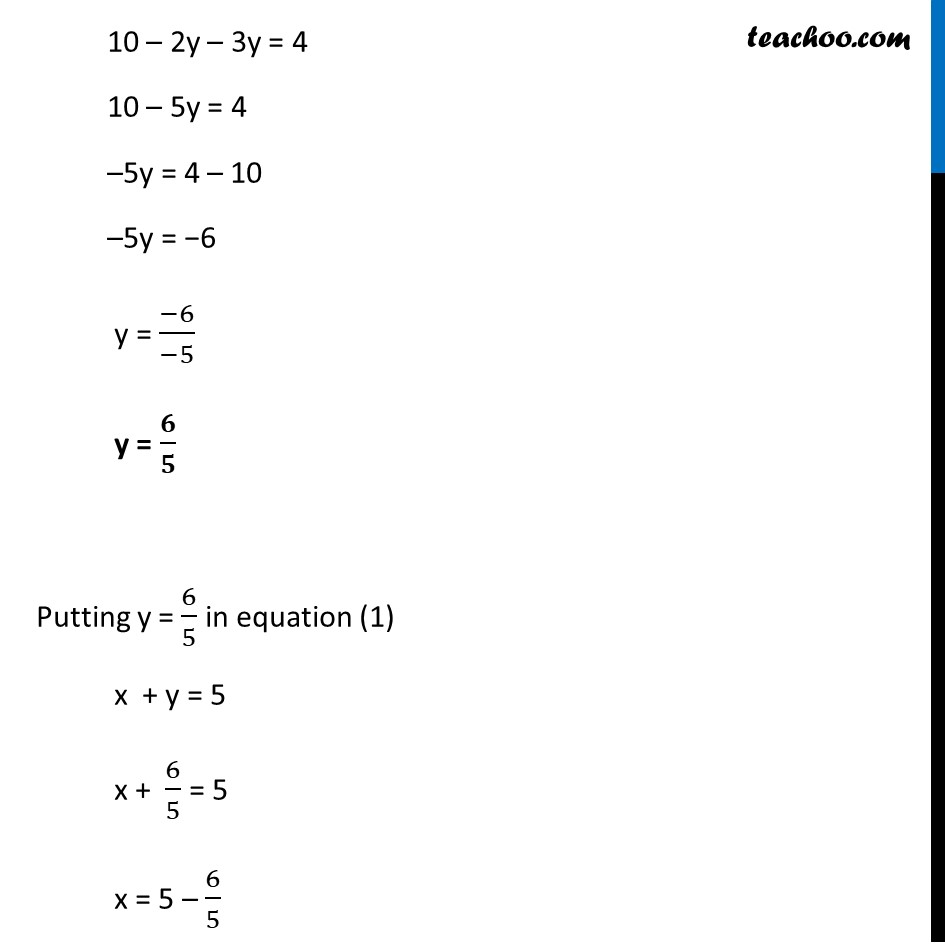
Transcript Ex 34, 1 (Elimination) Solve the following pair of linear equations by the elimination method and the substitution method (i) x y = 5 and 2x – 3y = 4 x y = 5 2x – 3y = 4 Multiplying equation (1) by 2 2(x y) = 2 × 5 2x 2y = 10 Solving (3) and (2) by Elimination –5y = –6 5y = 6 y = 𝟔/𝟓 Putting y = 6/5 in (1) x y = 5 x 6/5 = 5 x = 5 – 6/5 x = (5 × 5Section 41 Practice Test MATH110Section41PracticeTest 1 1(5,4 3x y=19 2x 3y=22 2(15 4x y=9 2x 4y=22 2 3 2x y=2 6x 3y=30 3 4 3x 4y=53 2x 2y=2 4 Section 41 Practice Test MATH110Section41PracticeTest 1Answer to Solve each system by multiplying 1 2x3y=5 x2y=1 2 3xy=2 8x2y=4 3 2x5y=22 10x3y=22 4 4x2y=14 7x3y=8 By signing up,
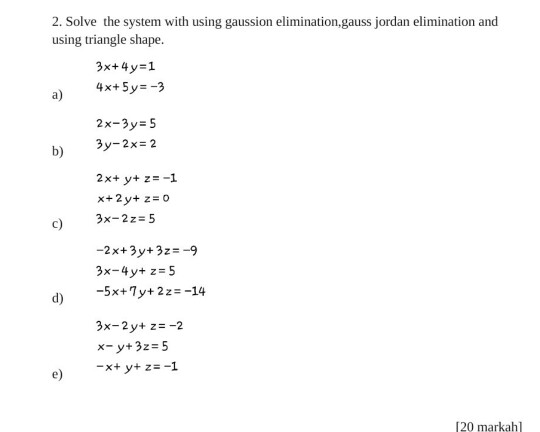



2 Solve The System With Using Gaussion Chegg Com




Show That X 2 Y 1 Is Not A Solution Of The System Of Simu
Solve 2x 1/10 3 2x/15 = x 2/6 Hence, find y, if 1/x 1/y 1 = 0 5x −5y = − 15 5x 3y = 1 0 − 8y = −16 Divide by −8 y = −16 −8 = 2 Substitute this is 1 x − (2) = −3 Add 2 to both sidesCEXAMPLE 3 Gaussian elimination Solve the system by using Gaussian elimination (a) 5 x 1 2y 2 2z 5 3 2x 1 3y 2 3z 5 1 24x 2 5y 1 5z 5 3 (b) 5 x 1 2y 2 2z 5 3 2x 1 3y 2 3z 5 1 24x 2 5y 1 5z 5 5 Solution (a) The following elementary operations lead to an echelon form, from which we find x, y,andz ~22!E 11E 2AE 2 5 x12y22z5 2y1 z5 24x25y15z5 3



Systems Of Linear Equations



Solving System Of Linear Equations Elimination Method Warmup
Graph 2x3y=5 2x 3y = 5 2 x 3 y = 5 Solve for y y Tap for more steps Subtract 2 x 2 x from both sides of the equation 3 y = 5 − 2 x 3 y = 5 2 x Divide each term by 3 3 and simplify Tap for more steps Divide each term in 3 y = 5 − 2 x 3 y = 5 2 x by 3 3 Use the elimination method 1) 3xy=1 5xy=9 2) 4x6y=24 4xy=10 3)2xy=3 x3y=16 4) 2x3y=7 3x4y=10 1 See answer mandaa97 is waiting for your help Add your answer and earn pointsSolve the following simultaneous equations2xy=5;




I Solve 2x 3y 17 2x 2 34 1 5




7x 3y 1 0
52 Factoring 3x2 10x 10 The first term is, 3x2 its coefficient is 3 The middle term is, 10x its coefficient is 10 The last term, "the constant", is 10 Step1 Multiply the coefficient of the first term by the constant 3 • 10 = 30 Step2 Find two factors of 30 whose sum equals the coefficient of the middle term, which is 10 30



Ssc Mathematics Chapter 4 Pair Of Linear Equations In Two Variables



1



7x 3y 1 0




Solve For X And Y Linear Equations Using The Elimination Method 2x 6y 2 5x 3y 3 And 9x 3y 5 9x 4y 6 Homeworklib
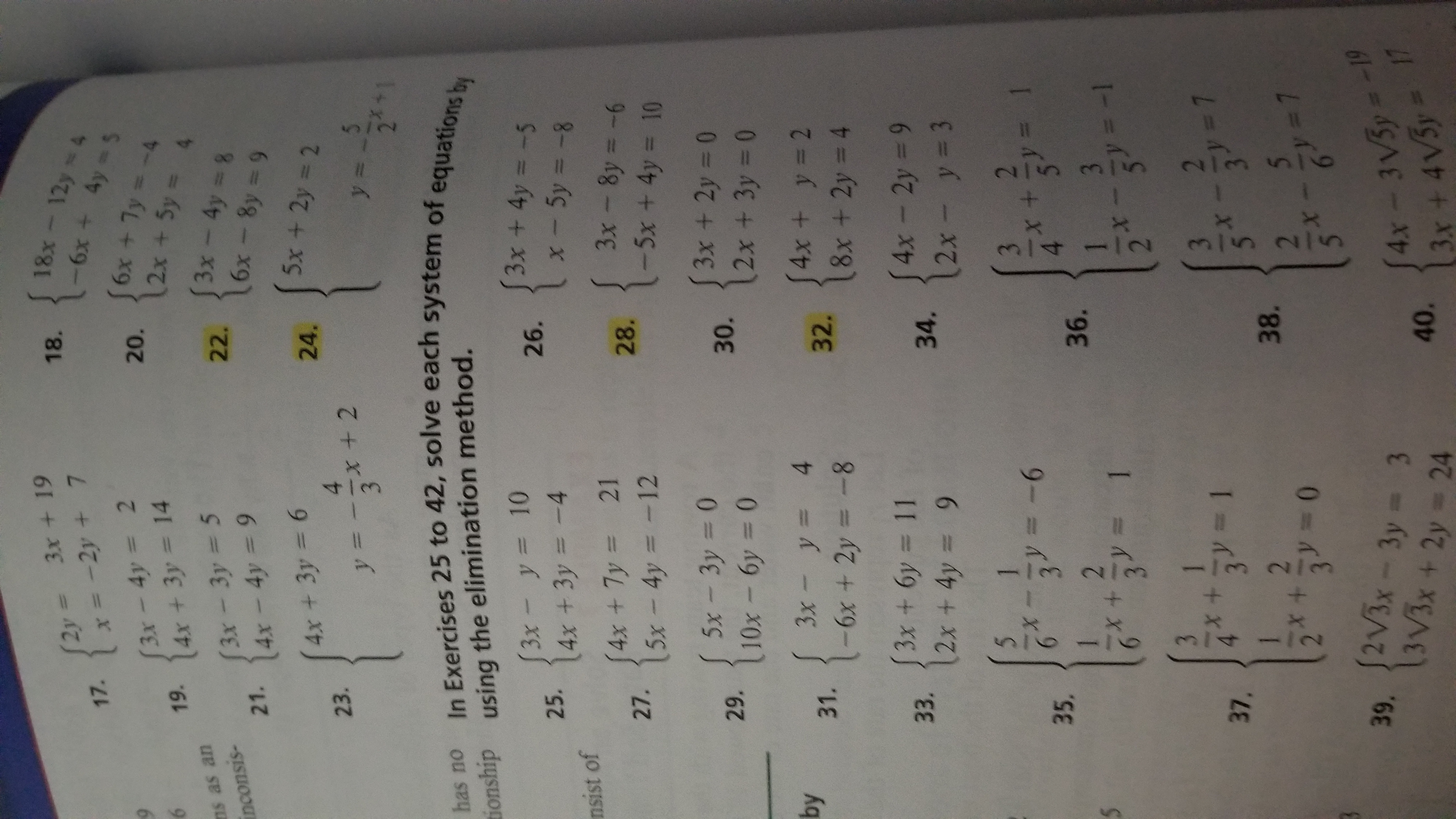



Answered 2y 3x 19 17 18x 12y 4 1 6x 4y 5 Bartleby




By Elimination Method Solve 3 2x 2 3y 5 5 X 3 Y 1 Brainly In




Rd Sharma Solutions For Class 10 Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 5 Avail Free Pdf



J19
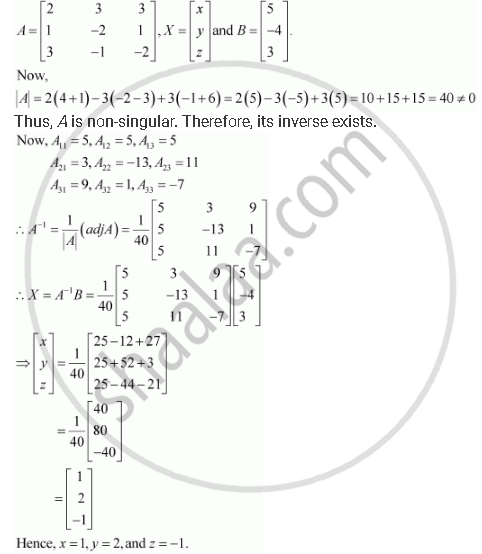



Solve System Of Linear Equations Using Matrix Method 2x 3y 3z 5 X 2y Z 4 3x Y 2z 3 Mathematics Shaalaa Com




7 X 5 2x Y 4 3y 5 5y 7 2 4x 3 6 18 5x Solve Using Cross Multiplication Brainly In
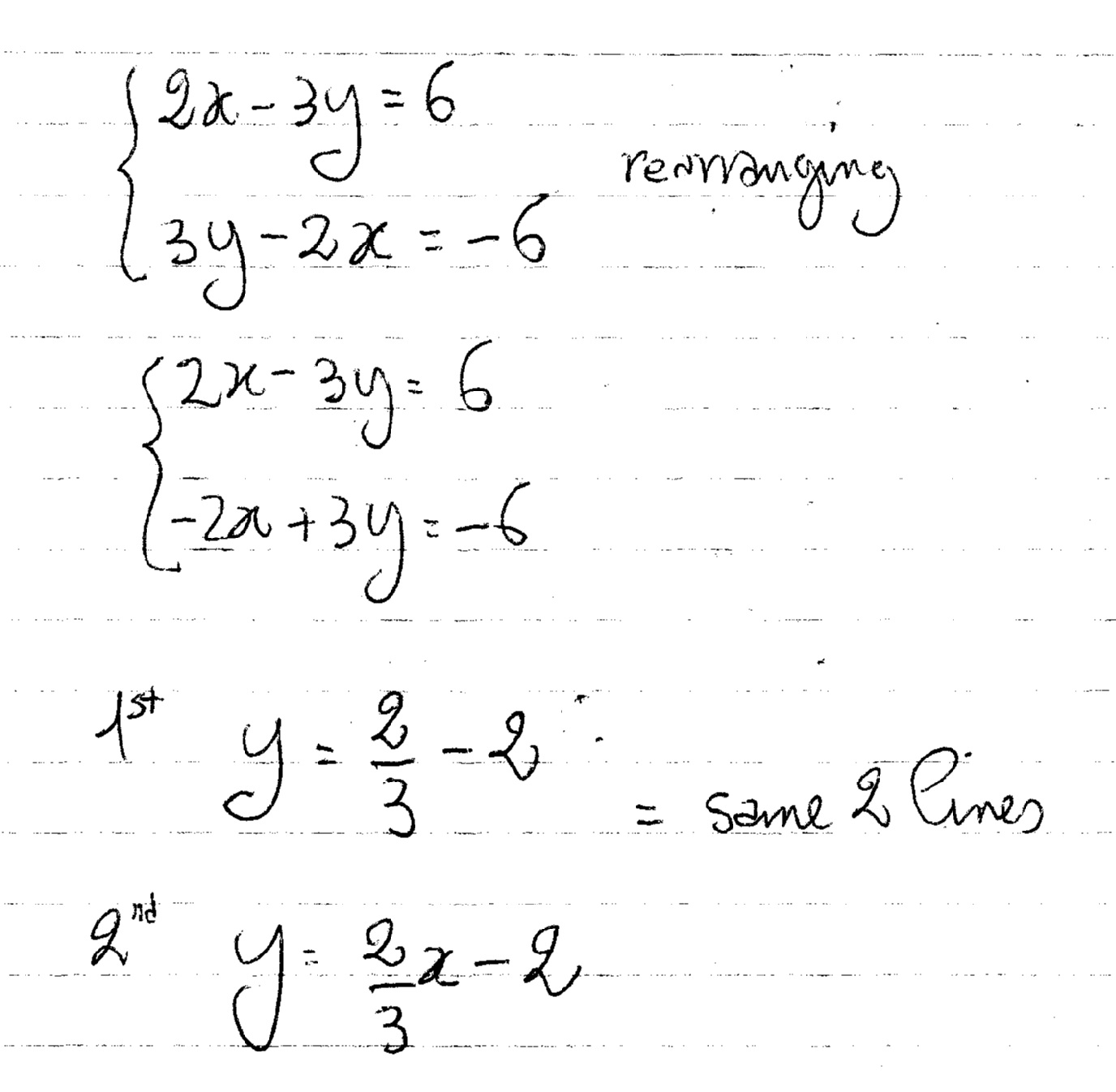



How Do You Solve The System Of Equations 2x 3y 6 And 3y 2x 6 Socratic



1



2x Y 2 X 3y 15 By Elimination Method




5 X 3 Y 1 3 2x 2 3y 5 Brainly In
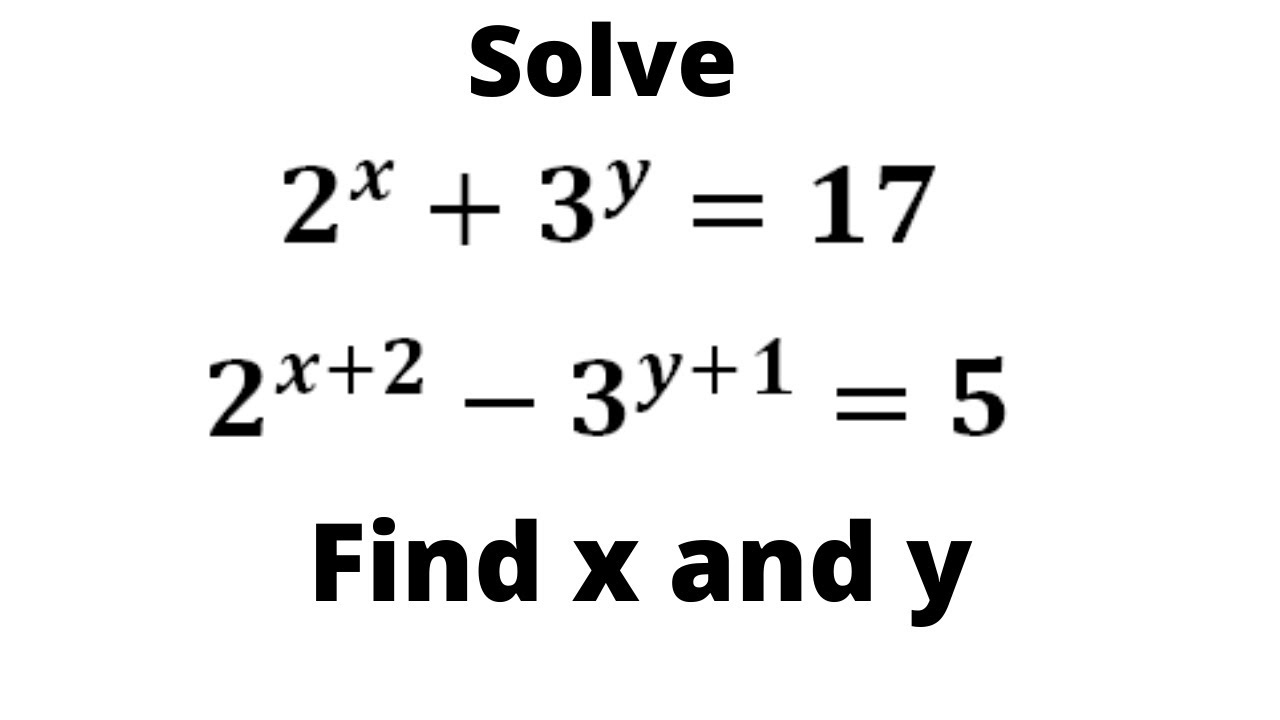



Solve 2 X 3 Y 17 2 X 2 3 Y 1 5 Find X And Y Youtube
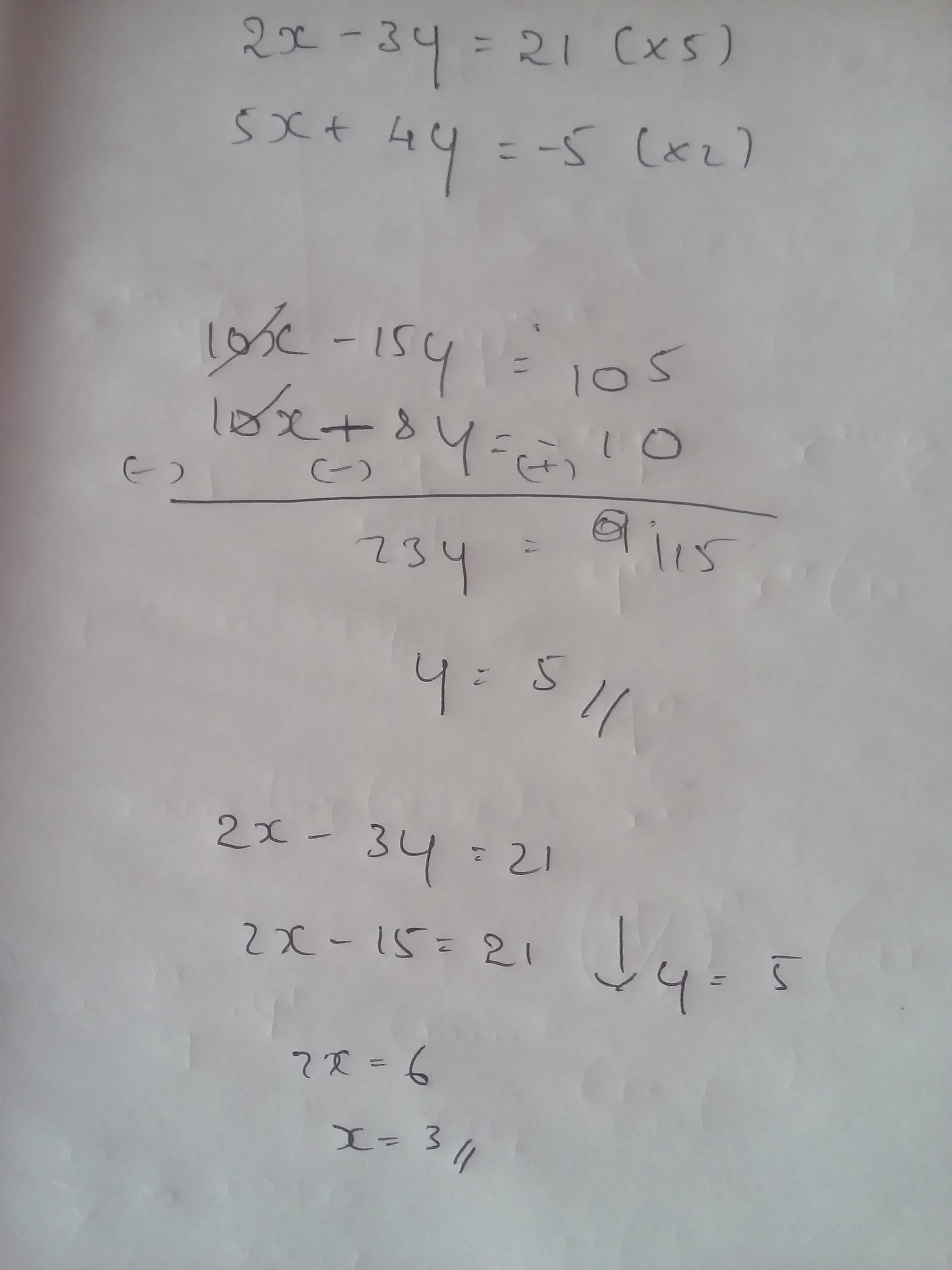



How Do You Solve 2x 3y 21 And 5x 4y 5 Socratic
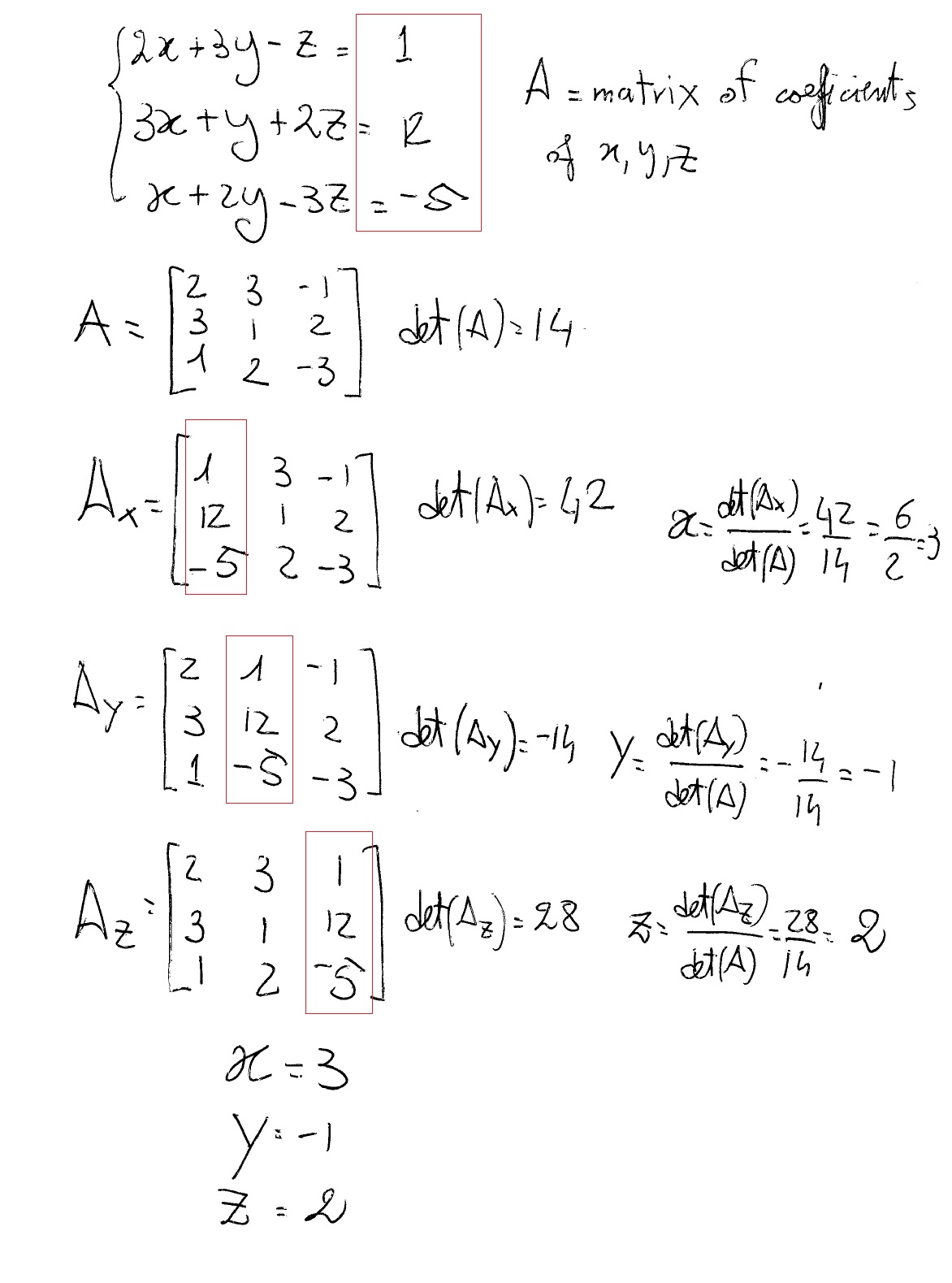



How Do You Solve 2x 3y Z 1 3x Y 2z 12 And X 2y 3z 5 Socratic



Solve The Following Simultaneous Equations 5x 3y 8 3x Y 2 Sarthaks Econnect Largest Online Education Community




3x 2 5y 3 2 And X 3 Y 2 13 6 Solve Using Substitution Method Youtube




X Y 5 And 2x 3y 4 Solve By Elimination Method Brainly In




1 Solve The Following Systems Of Linear Equations Chegg Com




Warm Up Simplify Each Expression 1 3x 2y 5x 2y Ppt Video Online Download
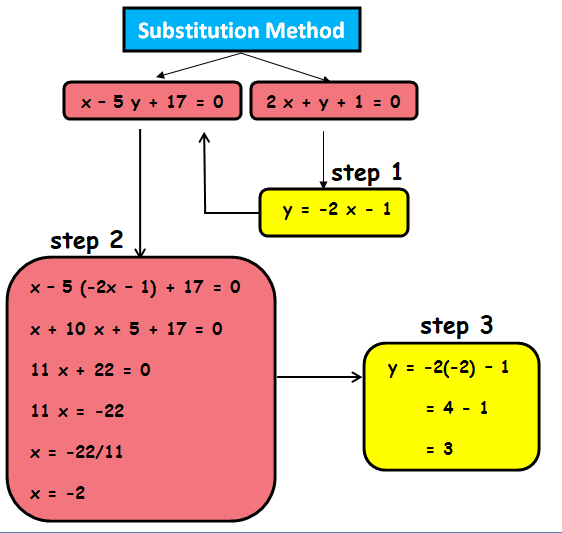



Substitution Method Examples




Answers




Chapter 5 Review Exercises




3 2x 2 3y 5 1 5 X 3 Y 1 2 Question On Simultaneous Linear Equation Solve This By Reducible To Pair Of Equation Method And Cross Maths Simultaneous Linear Equations Meritnation Com




2x 3y 5 X 2y 3 0 How To Solve This Problem With Elimination Method Brainly In
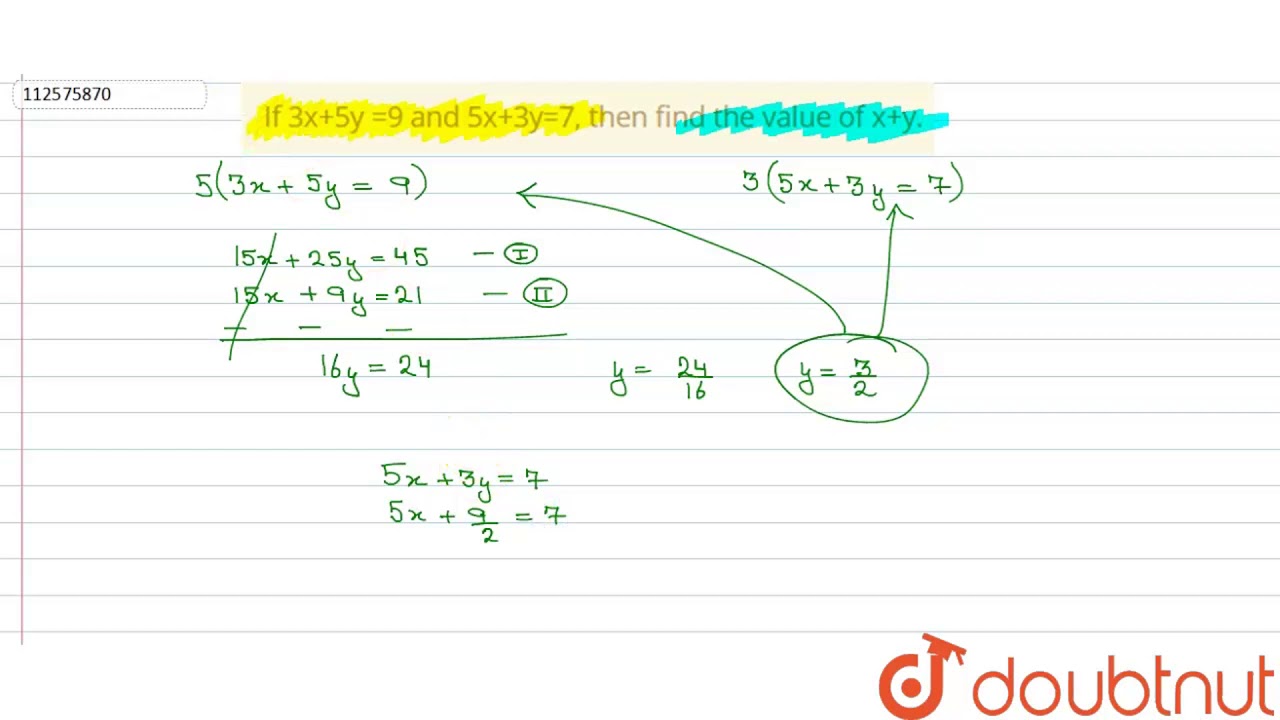



If 3x 5y 9 And 5x 3y 7 Then Find The Value Of X Y Youtube
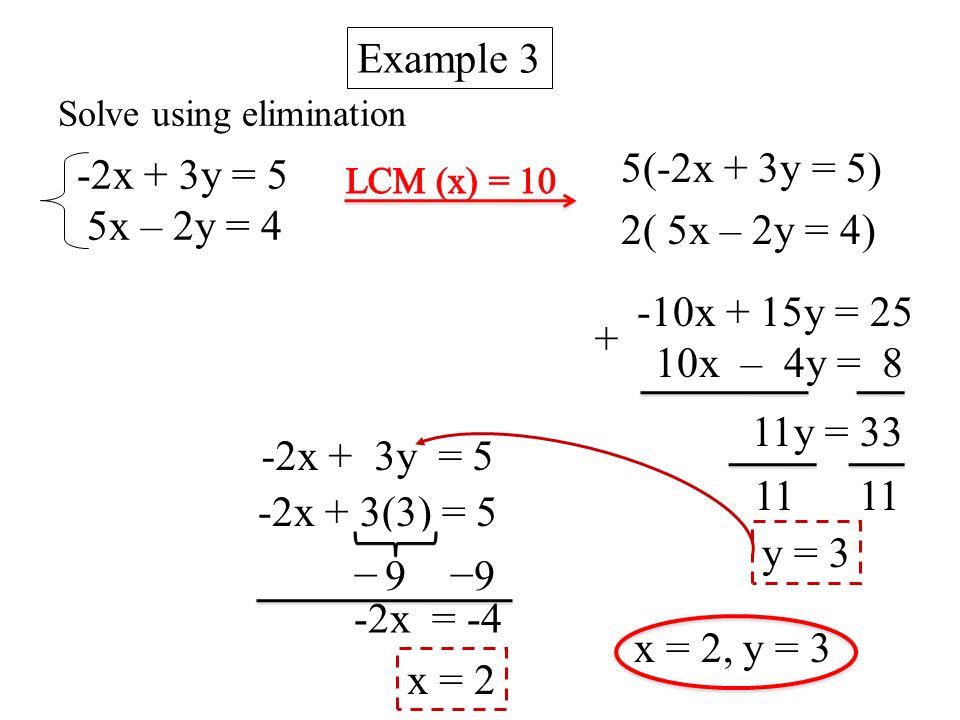



Ch 3 5 Elimination Multiplication Ppt Download
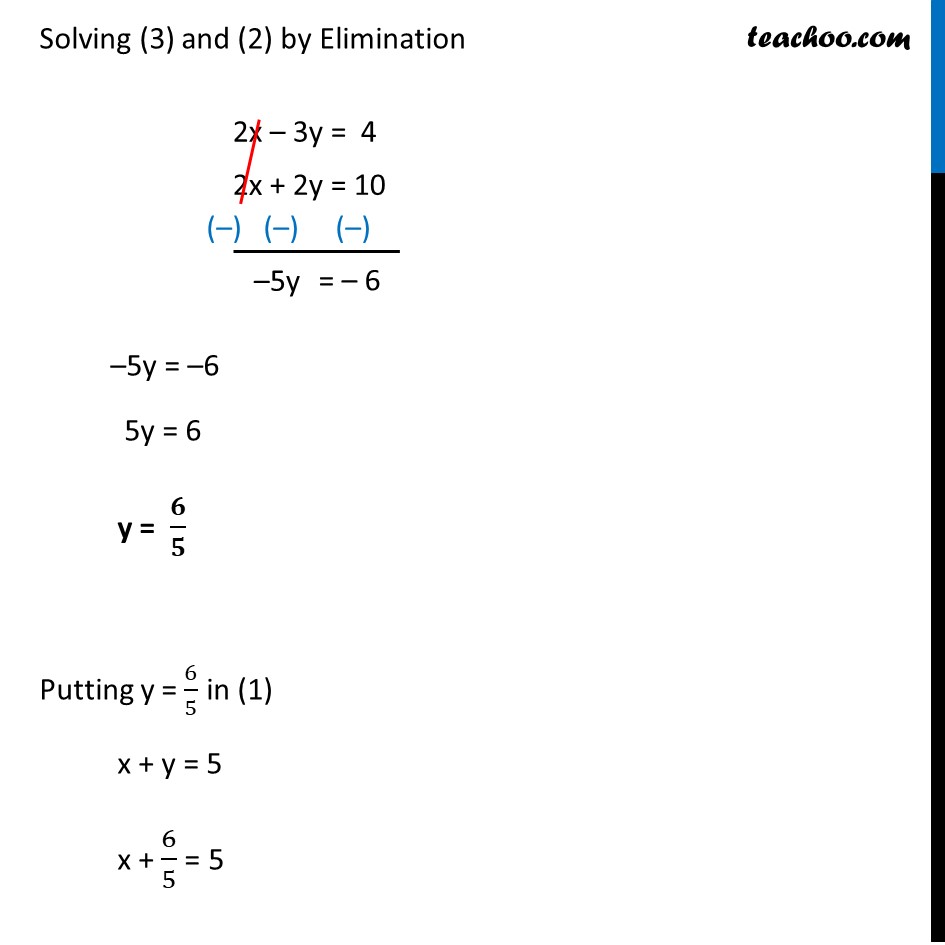



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y




4x 3y 5 5 2x Y 4 By Elimination Method Brainly In



How To Solve Using The Gauss Elimination Method Y Z 2 2x 3z 5 X Y Z 3 Quora
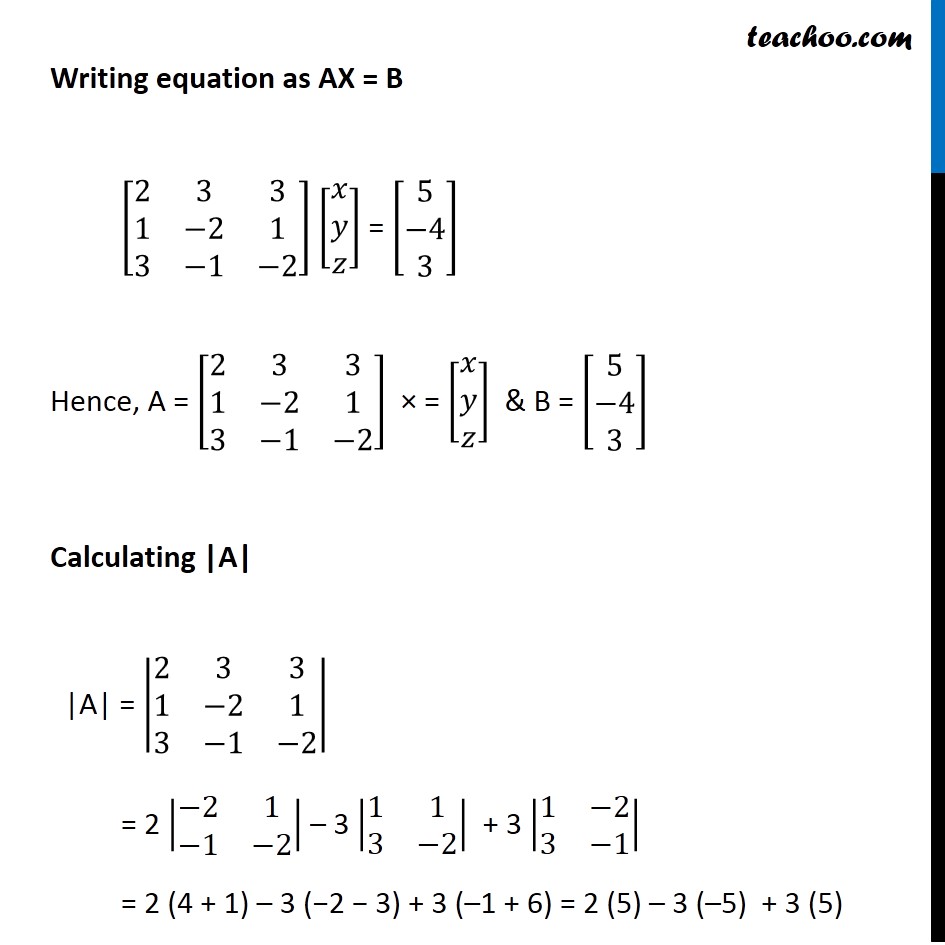



Ex 4 6 13 Solve Linear Equations Using Matrix Method Ex 4 6




Gseb Solutions For Class 10 Mathematics Pairs Of Linear Equations In Two Variables Cbse Tuts




7x 3y 1 0




3x 4y 10 4x 3y 5 Youtube




For What Value A And B The Following System Of Linear Equations Have An Infinite Number Of Solutions 2a 1 X 3y 5 3x B 2 Y 3 Mathematics Topperlearning Com Jafl8e



Q Tbn And9gcsiggdhv5fw1mvrwy6npui8oe9vjy7ffnulpwxqmdm604bzyzap Usqp Cau




Solve For X And Y 1 7x 1 6y 3 1 2x 1 3y 5 Brainly In




What Is The Solution For An Equation Of A Line Passing Through The Point Of Intersection Of 2x 3y 5 0 And 7x 5y 2 0 And Parallel To The Lines 2x 3y 14 0 Quora




Solve 2 X 2 3y 1 6 And 3 X 2 Y 0 And Hence Find A For
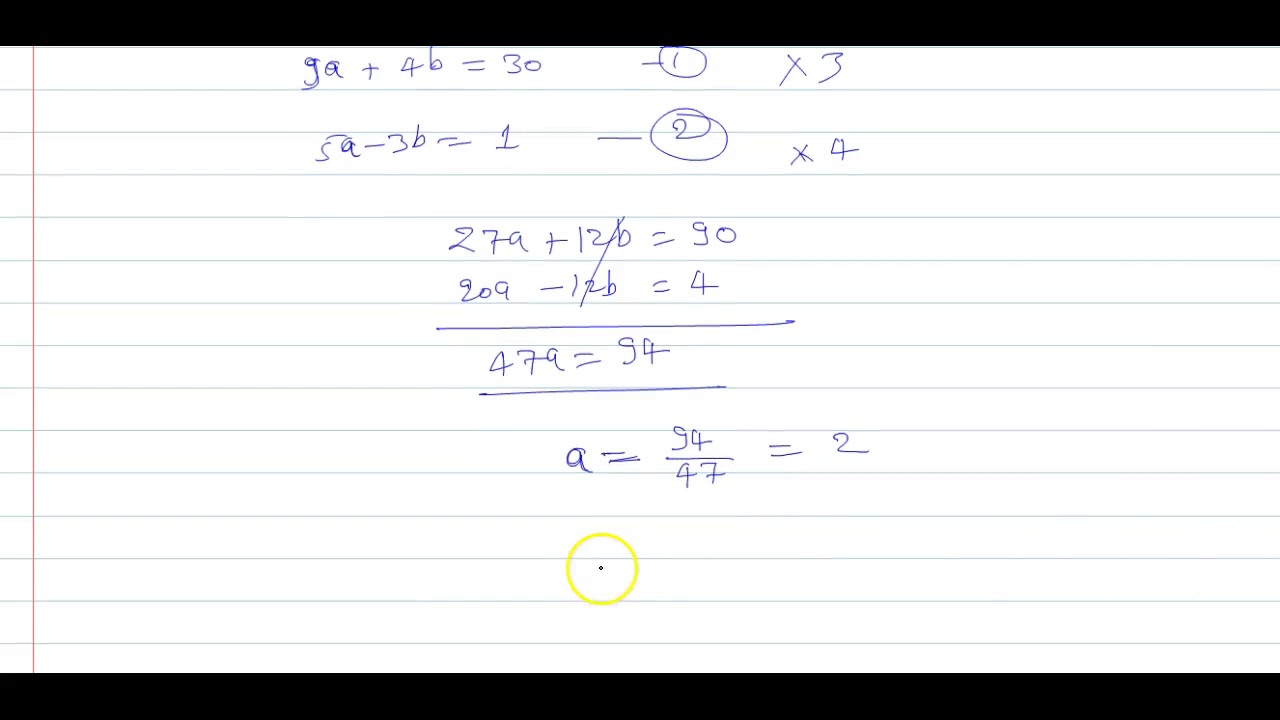



3 2x 2 3y 5 5 X 3 Y 1 Solve For X And Y Youtube
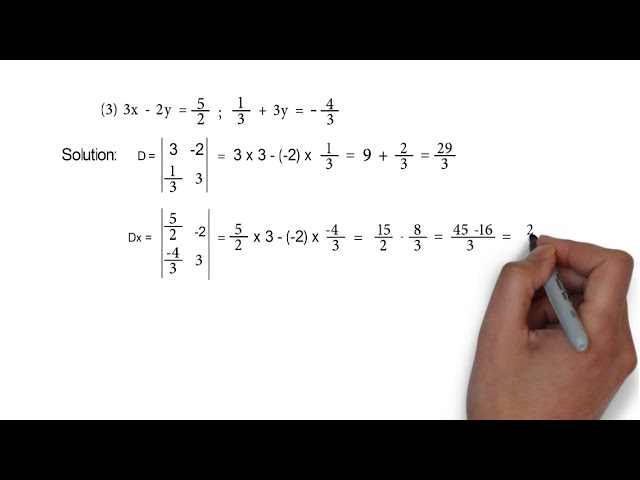



3x 2y 5 2 And 1 3 3y 4 3 Problem Set 1 Q5 3 Linear Equation In Two Variables Youtube
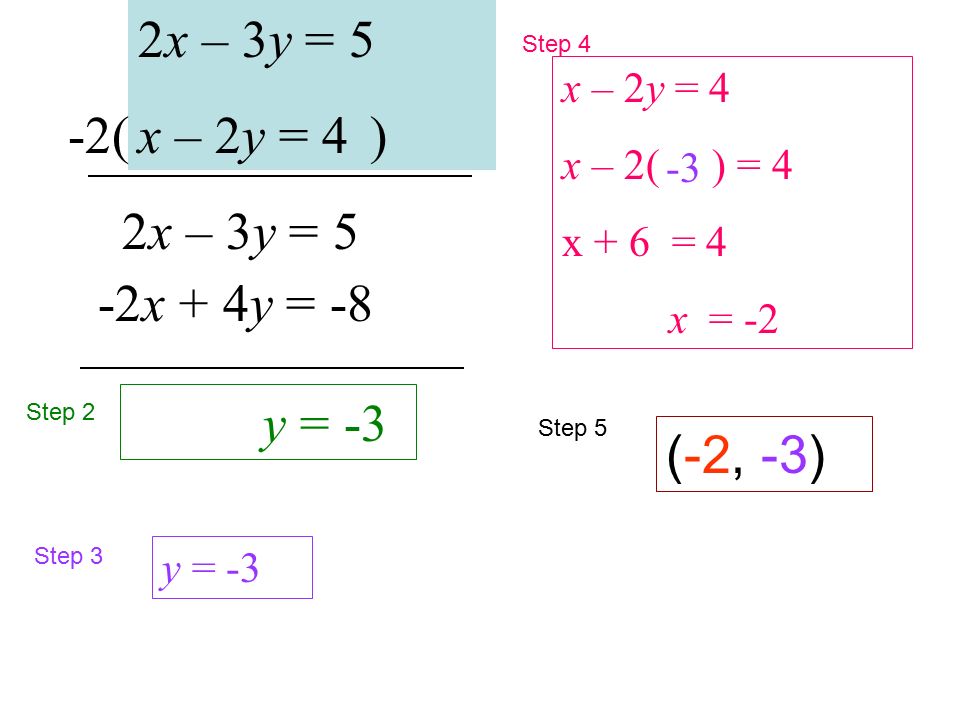



Elimination Method Day 2 Today S Objective I Can Solve A System Using Elimination Ppt Download




2 Using The Elimination Method Solve Each Of Li Gauthmath




5x 3y 1 Amp 2x 5y 19 By Elimination Meethod Brainly In
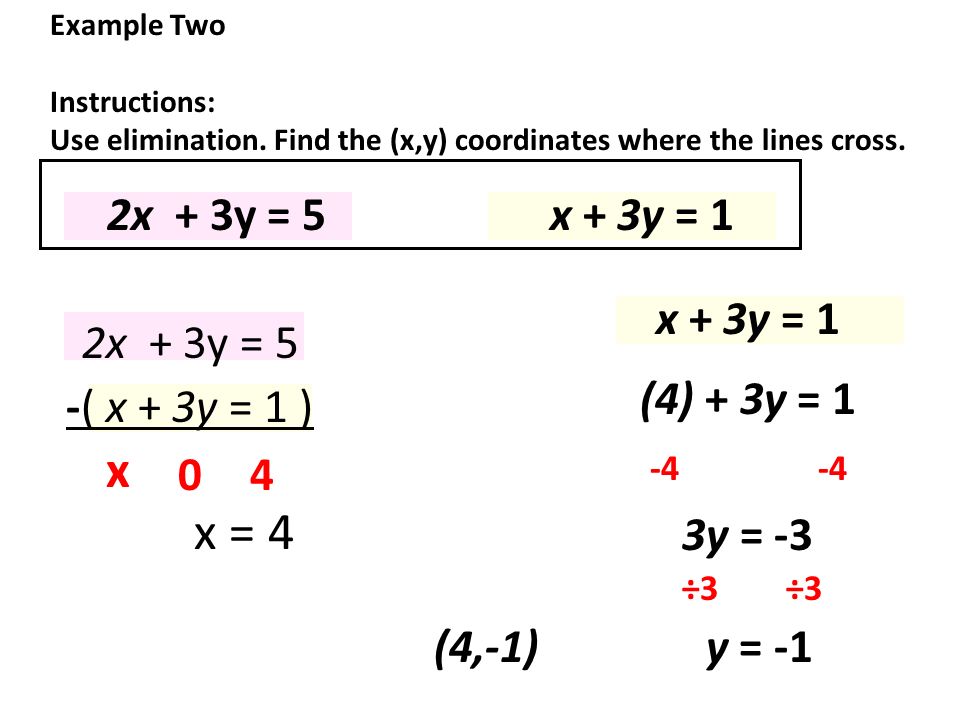



Vocabulary Chapter Section Topic Simultaneous Linear Equations Ppt Download




Bvvwyzsp2zsvdm




X 3y 5 3x Y 5 Brainly In
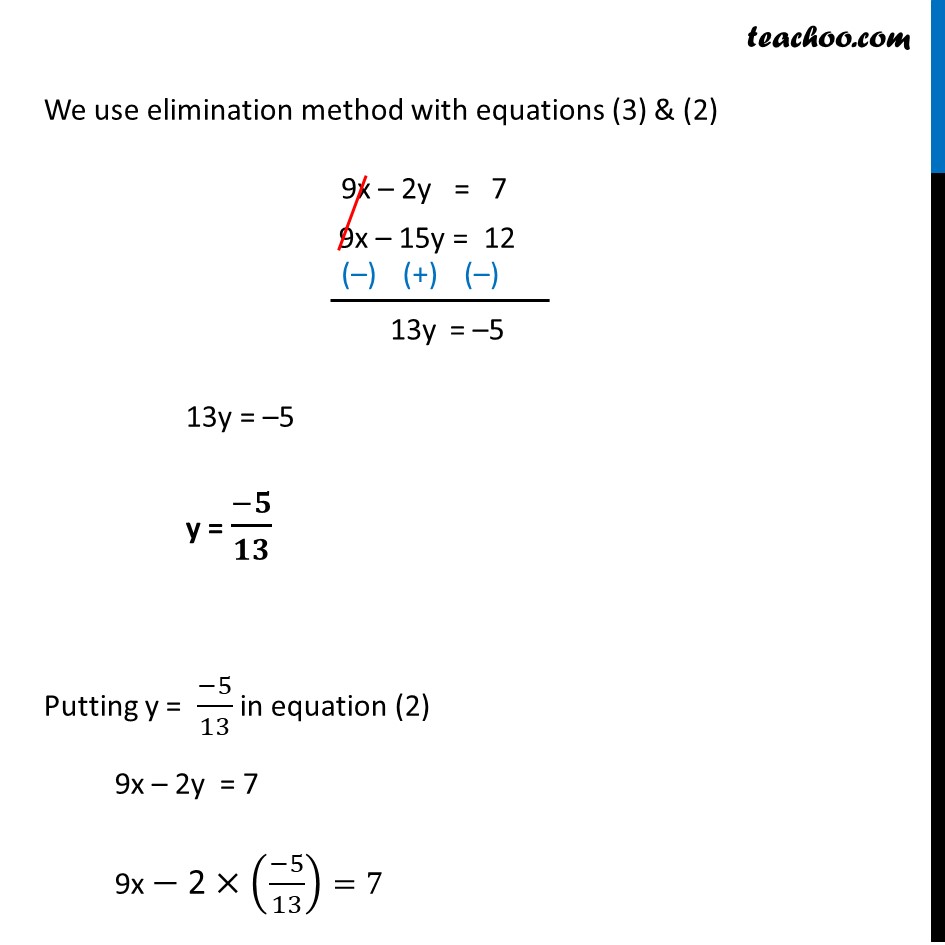



Ex 3 4 1 Solve By Elimination And Substitution I X Y 5 2x 3y
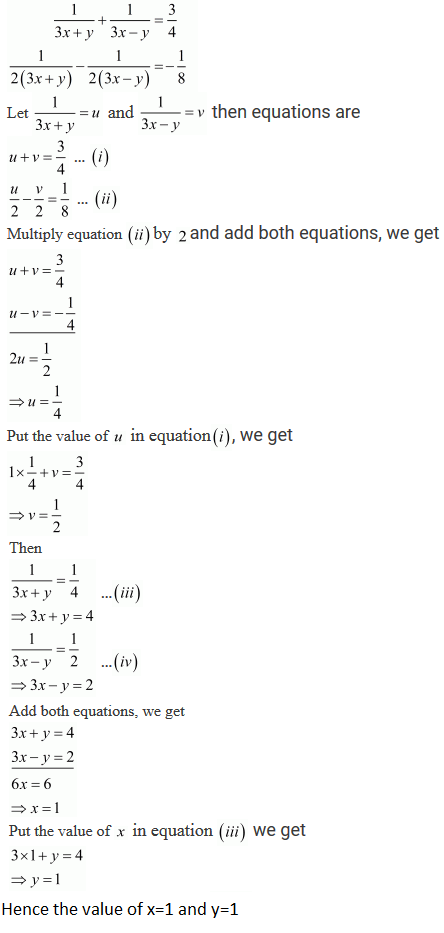



R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 3




Systems Of Equations With Elimination X 2y 6 4x 2y 14 Video Khan Academy




Write The Augmented Matrix For Each System Do Not Chegg Com




2x Y 2 3x 4y 6 10 9 4x Y 2x 3y In Problems Chegg Com




6 2 Solving Systems Of Linear Equations 455 We Have Chegg Com



最も好ましい 5 X 3 Y 1 3 2x 2 3y 5 By Substitution Method




Solve By Elimination Method X Y 5 2x 3y 5 Brainly In




Answered Exercise Set 9 1 S2y3d X 2y 7 Zx Bartleby




5x 3y 8 0 2x 3y 5 0 In Substitution Mothod Brainly In




X 2y 7 2x 3y 5 Novocom Top
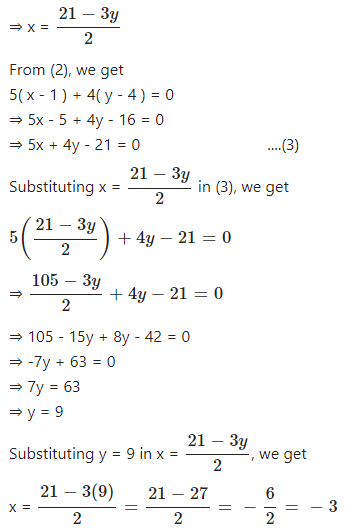



Simultaneous Linear Equations Class 9th Concise Selina Icse Maths Icsehelp



Solve The Following Systems Of Equations 1 7x 1 6y 3 1 2x 1 3y 5 Sarthaks Econnect Largest Online Education Community




Test The Consistency Of The System X Y 2z 1 X Y Z 3 X 3y 3z 1




Solve The Pair Of Linear Equations By Cross Multiplication Method 2 2x Y 5 3 X 3y 1 0 Brainly In




無料ダウンロード 2 X 2 Y 1 2 3x 2 3y 7 2 X 2 Y 1 2 3x 2 3y 7




What Is The Solution For An Equation Of A Line Passing Through The Point Of Intersection Of 2x 3y 5 0 And 7x 5y 2 0 And Parallel To The Lines 2x 3y 14 0 Quora




Systems Of Equations With Elimination 3y 4x 11 Y 2x 13 Video Khan Academy
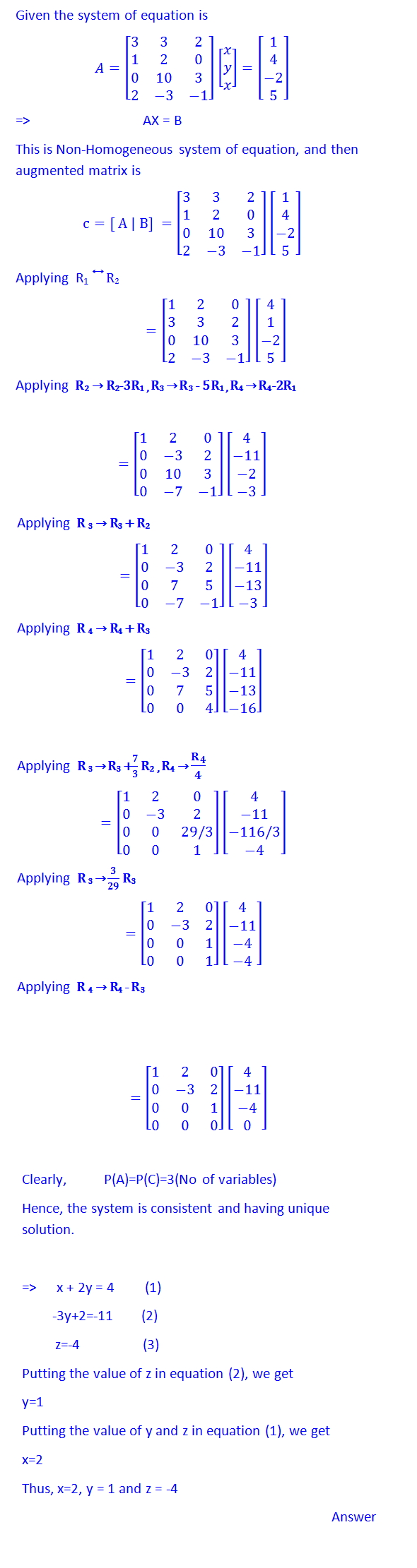



Solve The System Of Equations 3x 3y 2z 1 X 2y 4 10y 3z 2 And 2x 3y Z 5 Answer Mathematics 1 Question Answer Collection



7x 3y 1 0



How To Solve The Following Simultaneous Equations By Elimination Method A 2x 3y 12 X Y 1 B 3x Y 10 C X 3y 1 3x 2y 4 0 D 5 Quora



1



Mfm2p
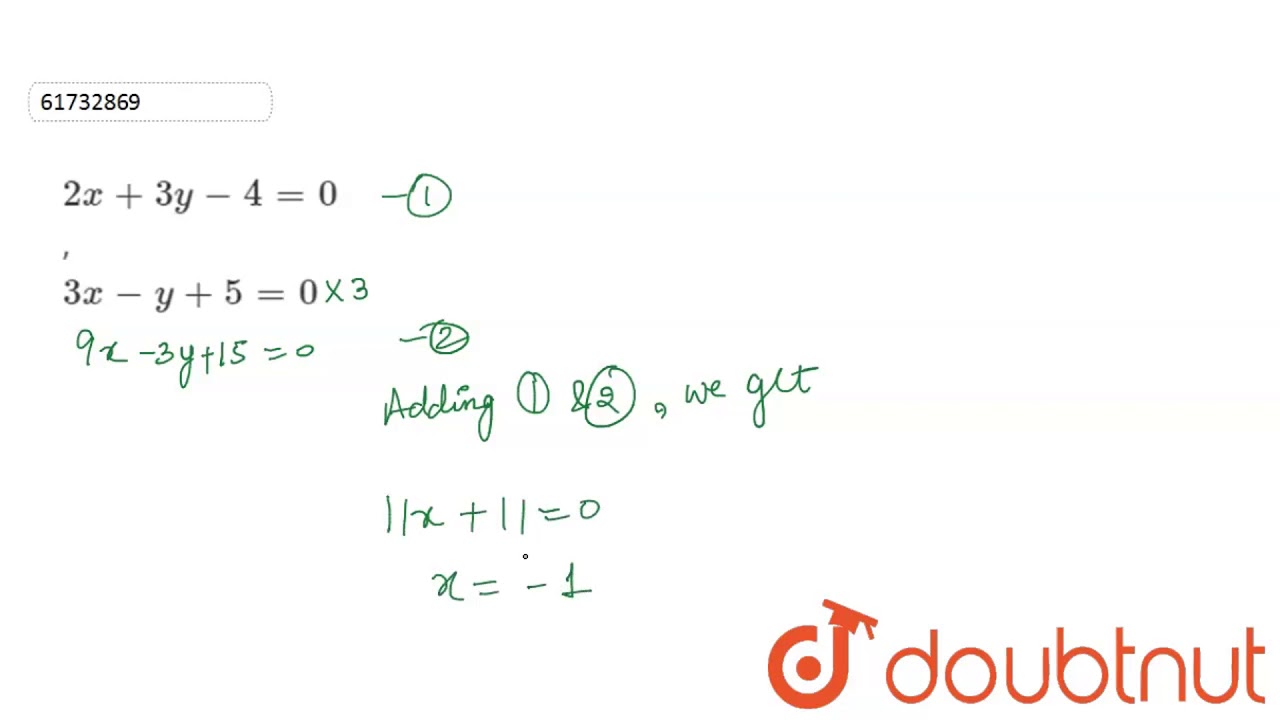



2x 3y 4 0 3x Y 5 0 Youtube




Solve By Matrix Method 2x 3y 3z 5 X 2y Z 4 3x Y 2z 3
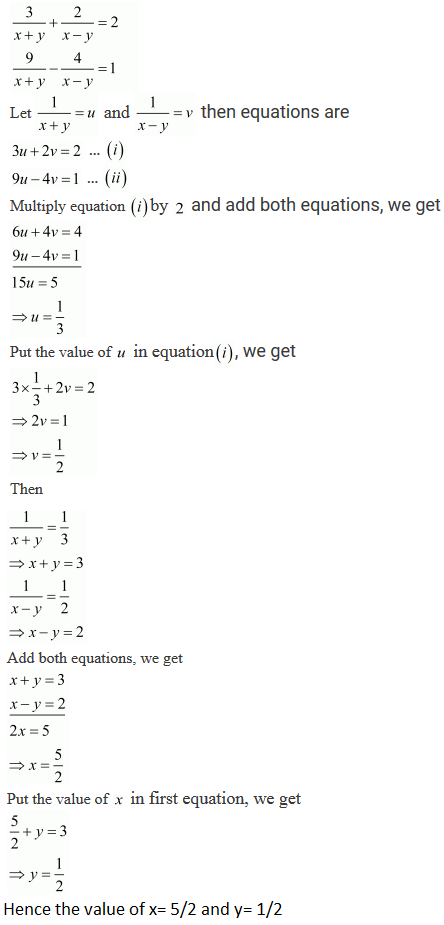



R D Sharma Solutions Class 10th Ch 3 Pair Of Linear Equations In Two Variables Exercise 3 3



Solve For X And Y 5 X 3 Y 1 3 2x 2 3y 5 Sarthaks Econnect Largest Online Education Community



0 件のコメント:
コメントを投稿